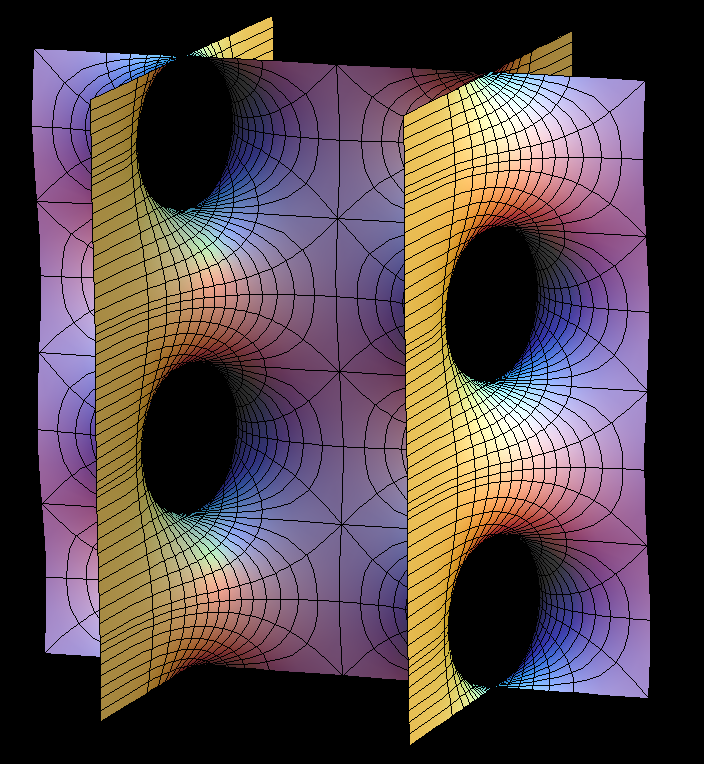
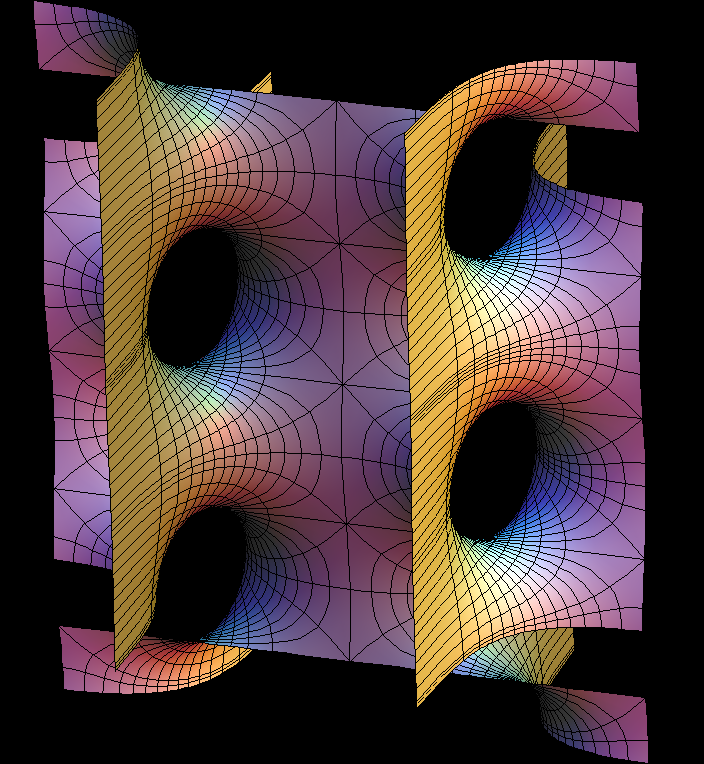
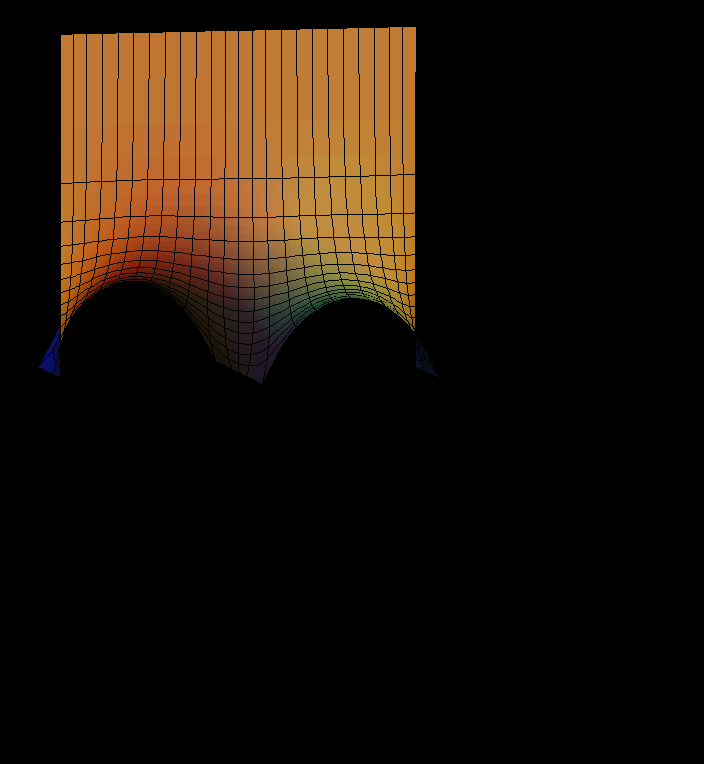
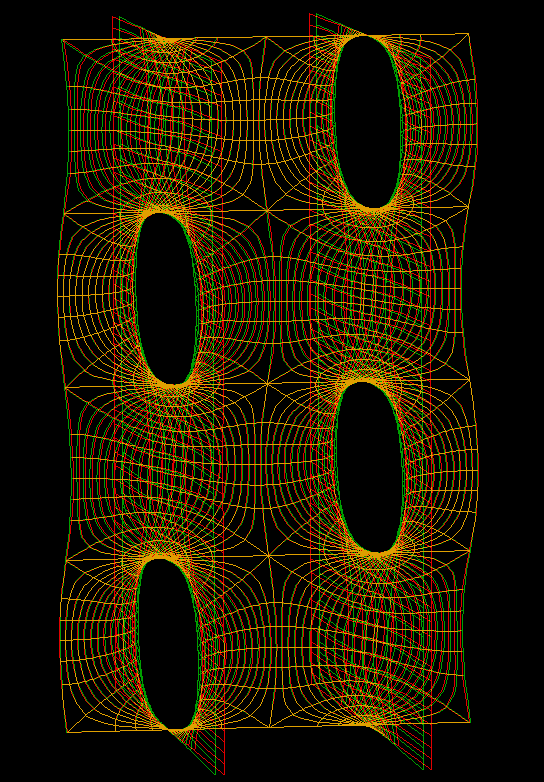
These families are parametrized by 4-punctured rectangular tori; they and their conjugates are embedded. We therefore suggest the associate family morphing, and also morphing of the modulus, bb, (0 < bb < 0.5) of the rectangular Torus, which changes the size of the visible holes.
For the visual appearance of these surfaces it is particularly important that the punctures are centers of polar coordinate lines. Formulas are taken from [K1] or [K2]. The Gauss maps for these surfaces are degree 2 elliptic functions. The cases shown are particularly symmetric, the zeros and poles of the Gauss map are half-period points and the punctures are there. In the Jd-case the diagonal of the rectangular fundamental domain joins the two zeros, and in the Je-case it joins a zero and a pole of the Gauss map.
Under suitable choices of the modulus of the Torus these surfaces look like a fence of Scherk Saddle Towers - with a vertical straight line (Je), respectively a planar symmetry line (Jd), separating these towers. The conjugate surfaces look qualitatively the same in the Jd-cases and like a checkerboard array of horizontal handles between vertical planes in the Je-cases.
For a discussion of techniques for creating minimal surfaces with various qualitative features by appropriate choices of Weierstrass data, see either [KWH], or pages 192--217 of [DHKW].