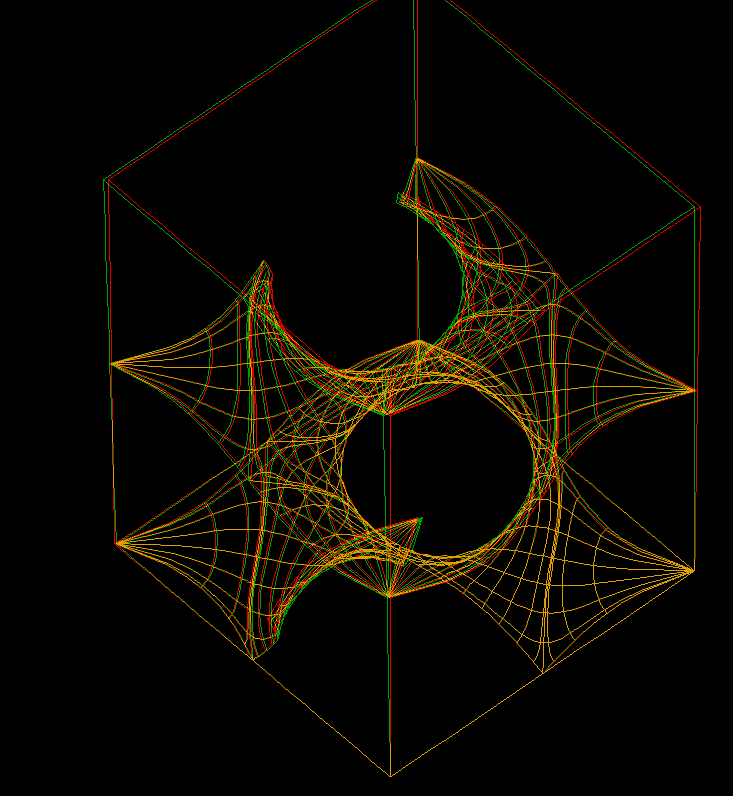
The Lidinoid and the Gyroid are the only known triply periodic embedded minimal surfaces which are NOT cut by straight symmetry lines and/or planar symmetry curves into simple pieces. These symmetries allow in all cases but the Gyroid and the Lidinoid to find a fundamental domain of the surface (for the translation symmetries) which is exactly the intersection of the surface with a cristallographic cell for its lattice of translations. This is an enormous help for visualizing the surfaces.

The Lidinoid is a member of the associate family of one of the Schwarz H Family Surfaces.
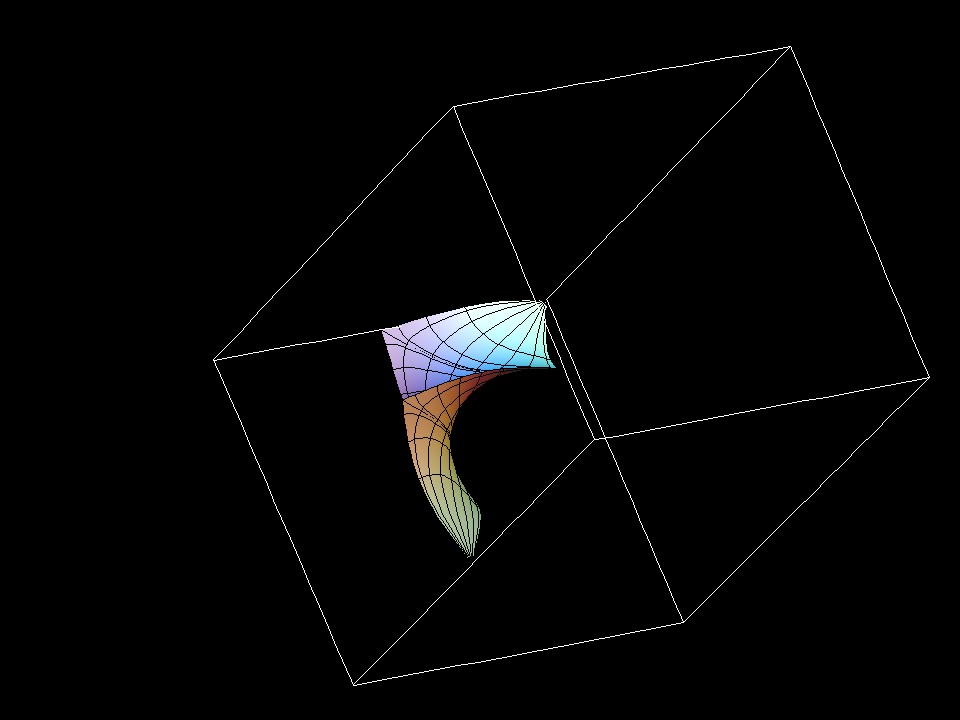
The animation begins with a translational fundamental domain of this H-surface and ends with the corresponding piece of the Lidinoid, together with its cristallographic cell. Note that the edges of the cell indicate translation symmetries of the Lidinoid. Similarly, the white segments in the other images are also translation symmetries of those surfaces, but (except for the H-surface) they are not part of a lattice.
Associate family deformations do not change the length of curves on the surfaces and therefore also do not change the Gauss curvature. Moreover, the surface normals at corresponding points stay parallel during the deformation. One says: All surfaces of the associate family have the same Gauss map.
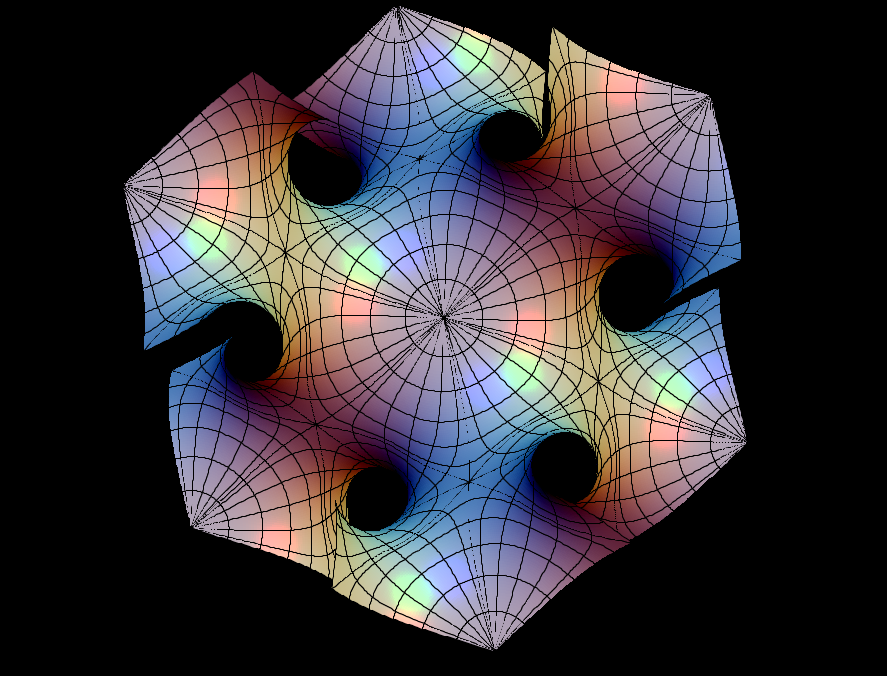
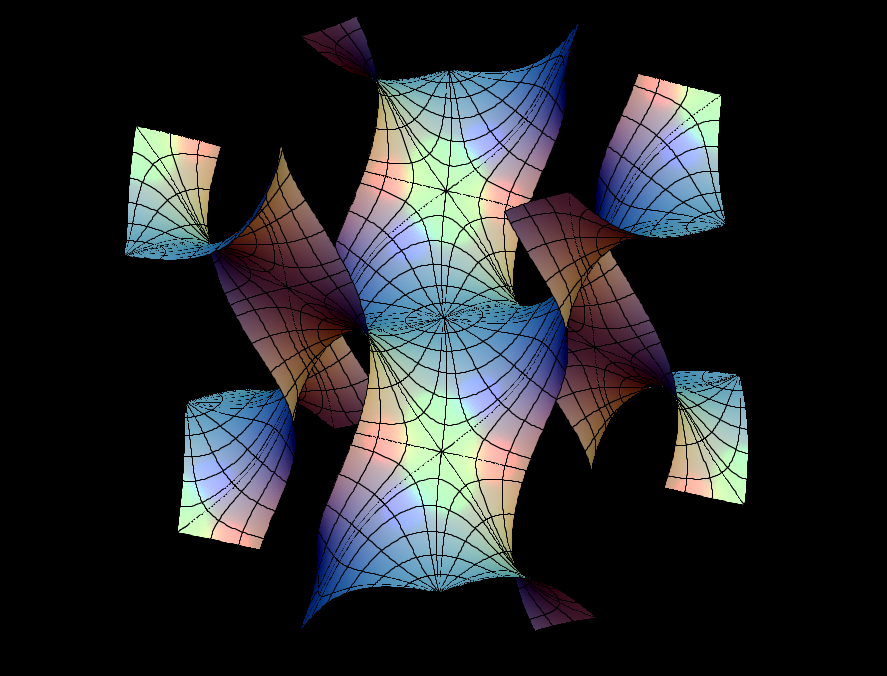
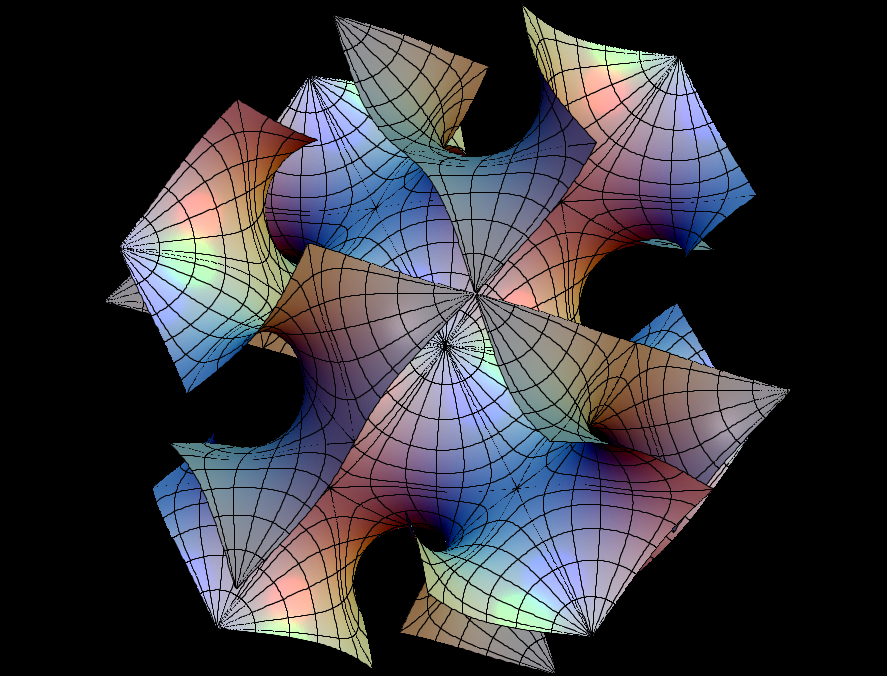
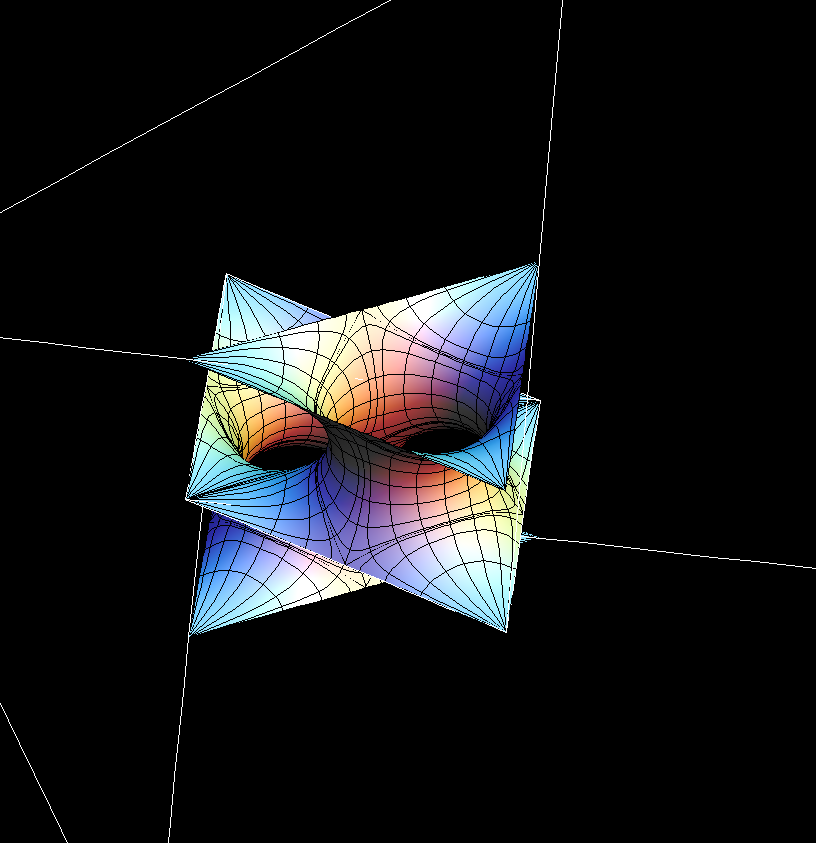
Our grid for the Lidinoid has parts which look like polar centers. These fill what we will call curved hexagons. The centers of these hexagons are symmetry points: reflection in them is a symmetry of the surface. The compositions of any two of these point reflections are translation symmetries of the surface. The surface normals at these centers are all parallel or antiparallel. We draw the Lidinoid with a fundamental cell that has one set of edges passing orthogonally through the centers of these hexagons. The other 8 edges of the fundamental cells meet at their midpoints the surface in hexagon vertices which are another kind of symmetry points: They are also centers of point symmetries, but at these points the surface is not as flat as in the middle of the hexagons. Finally there are 180 degree rotations around certain surface normals which are also symmetries of the surface; on the Schwarz-H-surface these normal symmetry points can easily be seen, namely where planar symmetry lines cross orthogonally. They are less obvious here. The image sequence shows the boundary of a fundamental cell to which more and more small pieces of the surface are added.
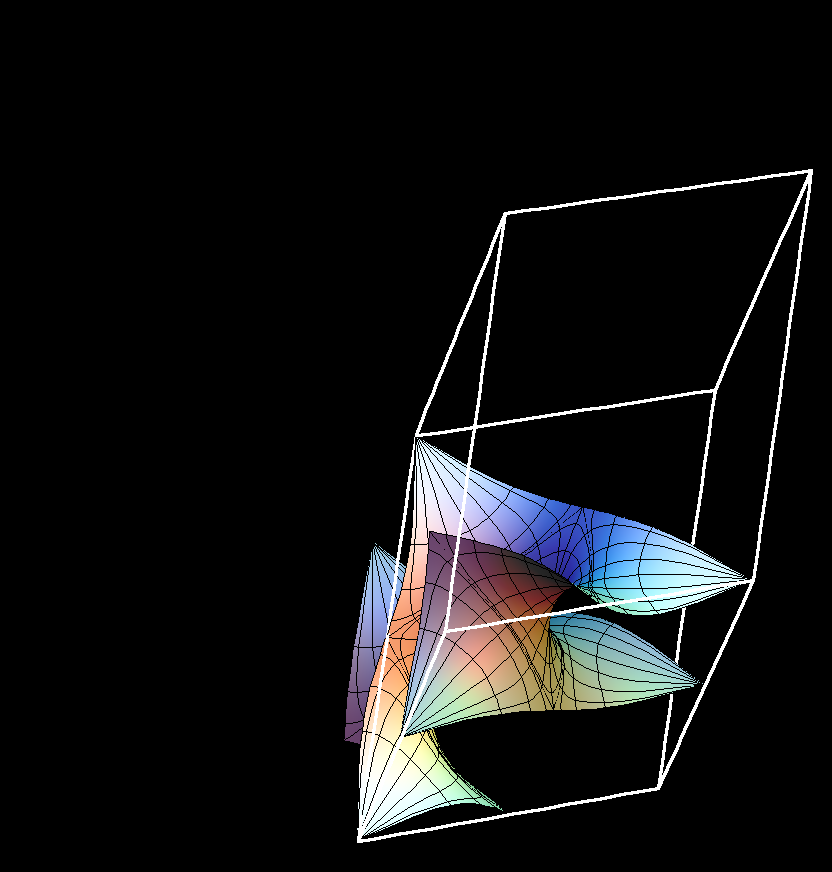
Again, the image sequence shows the same fundamental cell as before, but from a different direction. This causes the cell to look quite differently, but this is only our interpretation of the picture, the cell is really the same as before. Here we add larger surface pieces than before. Note that the hexagons are connected through their vertices only with hexagons whose normals point in the same direction. The parameterlines from the hexagon centers through the midpoints of its edges continue through the most strongly curved parts of the surface and end up in the hexagons above and below, whose normals point in the opposite direction. Note that the surface also has 120 degree rotation symmetries around the normals at the hexago centers.