The logarithmic spirals are defined by these equations:
c(t) = exp(growth*t) * [ cos(t), sin(t) ]
In complex notation: x(t)+i*y(t) = exp((growth+i)*t)
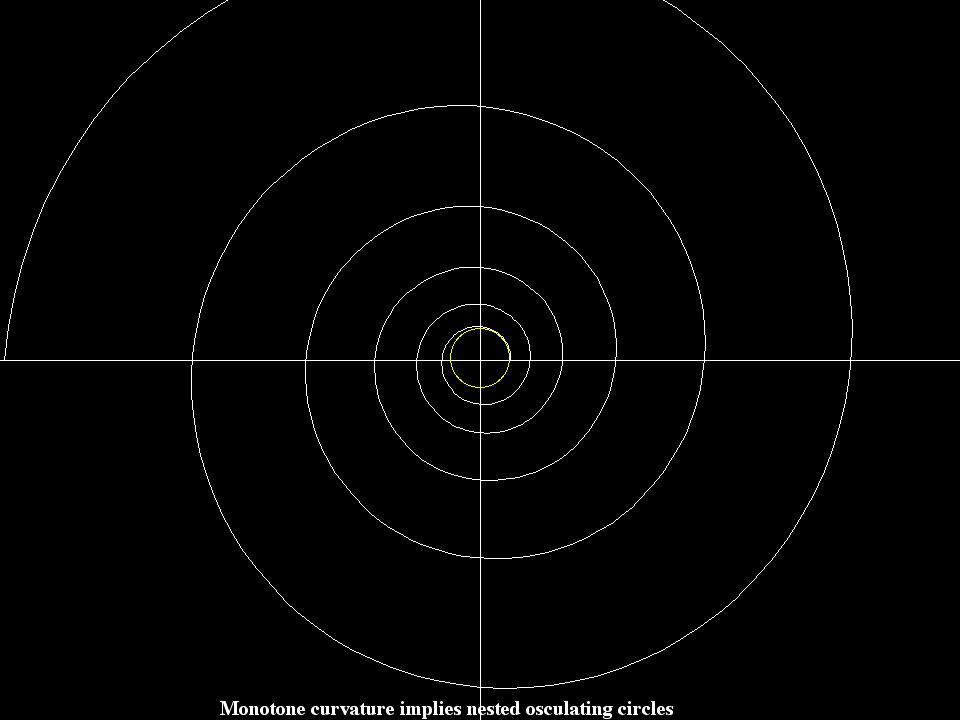
The spirals have monoton curvature functions,
therefore their osculating circles are nested.
c(t) = exp(growth*t) * [ cos(t), sin(t) ]
In complex notation: x(t)+i*y(t) = exp((growth+i)*t)
The spirals have monoton curvature functions,
therefore their osculating circles are nested.
At growth = 0.166 (also near 0.122, 0.276) the spiral is an autoevolute in the sense of W.Wunderlich, i.e. the centers of the osculating circles lie on the spiral.
Logarithmic_Spiral.pdfRelations to ODEs: The tangent vectors to the osculating circles define a vector field on the plane outside the origin. The circles and the spiral are integral curves of this vector field, so that uniqueness of ODE solutions fails. Hence: this vector field is not Lipschitz continous.
Any angular piece of this spiral is similar to any other piece of the same angular size