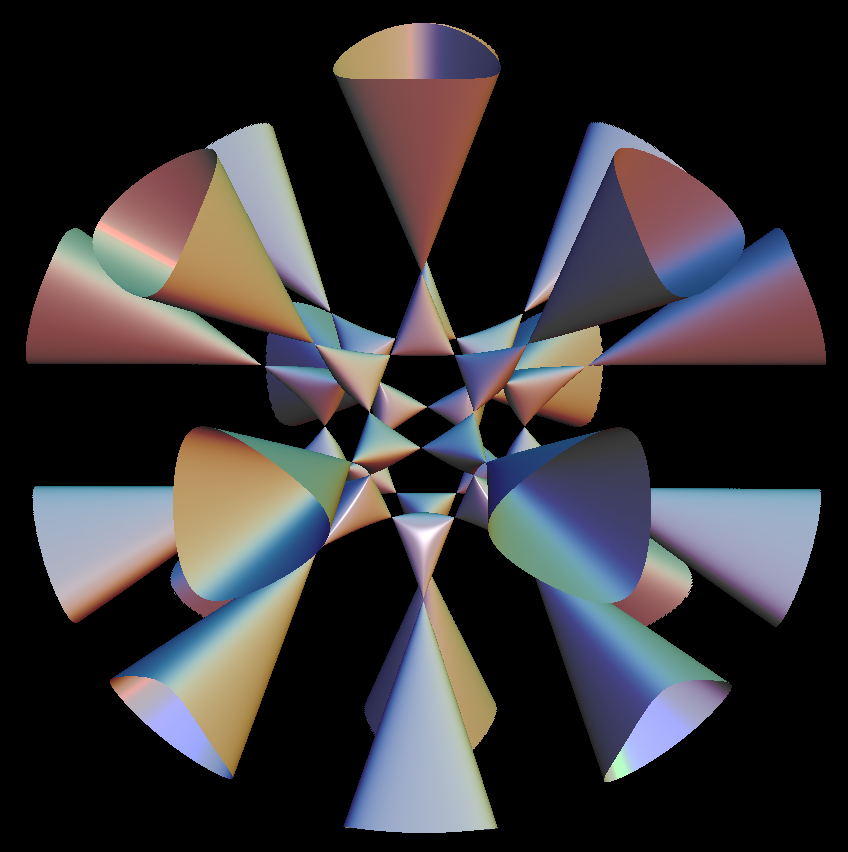

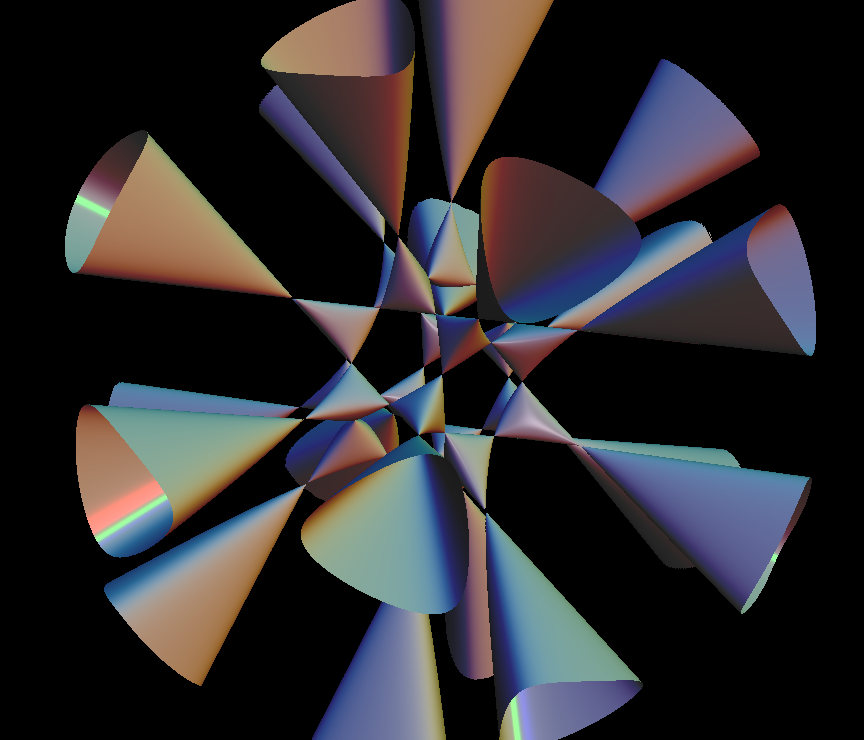
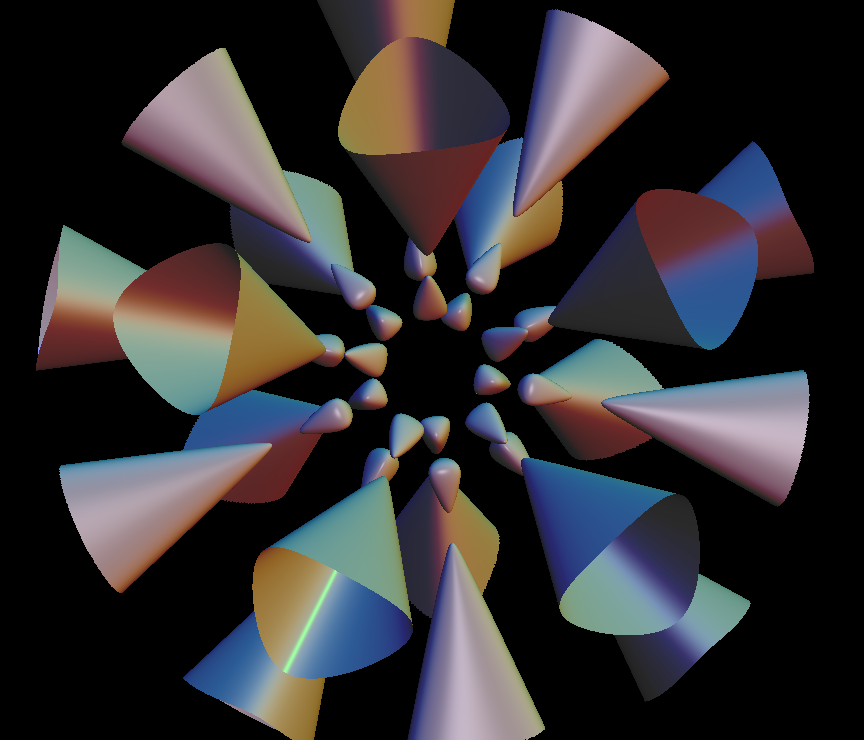
Morse Theory
This theory says:
If you have on a compact differentiable manifold M a differentiable function
f : M ⟶ R
such that at every critical point p (i.e. grad f(p) = 0 ) the (symmetric) matrix of second partial derivatives has no 0 eigenvalue, then f is called a Morse function.
The topology of the sublevels of f (i.e. f^{-1} ( [min(f), x] ) ) changes only if x is a critical value (i.e. there exists p with f(p) = x and grad f(p) = 0). The way the topology changes, is determined by the index of the matrix of second partial derivatives at the critical point p.
Our animation sequence shows an example of this change of topology.
For more detail, see Morse theory
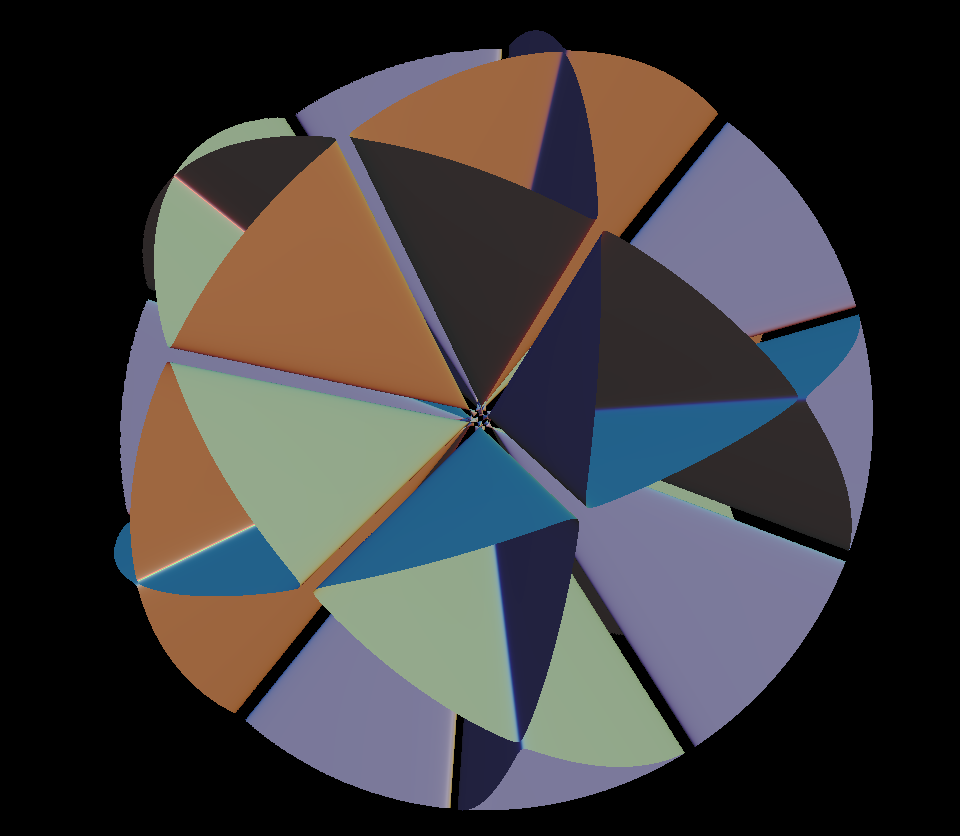
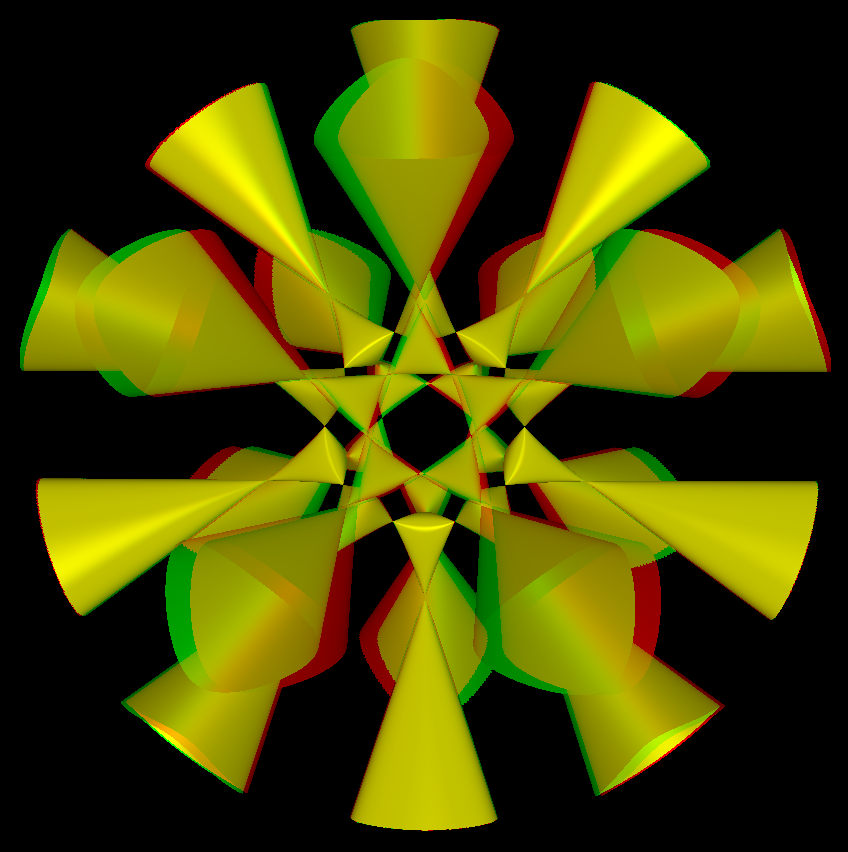
Barth Sextic is a algebraic surface of degree 6. Its formula is:
4*(ϕ^2*x^2-y^2)*(ϕ^2*y^2-z^2)*(ϕ^2*z^2-x^2)-(1+2*ϕ)*(x^2+y^2+z^2-w^2)^2 * w^2 == 0
where ϕ is the golden ratio (ϕ = (1 + Sqrt[5])/2 ≈ 1.61803) and w is the parameter.